In the late 17th century, Gottfried Wilhelm Leibniz discovered a beautiful series expansion of π/4 which only involves odd numbers. The identity can be derived from the derivative of the Arctangent \( \huge \frac{d}{dx}\arctan(x) = (1+x^2)^{-1}\). Based on this we have
$$\Large \begin{align} \frac{\pi}{4} = \arctan(1) &= \int_0^1 \frac{1}{1+x^2} \\
&=\int_0^1 1-x^2+x^4-x^6+\ldots\\
&=1-\frac{1}{3}+\frac{1}{5}-\frac{1}{7}+\ldots\end{align}$$
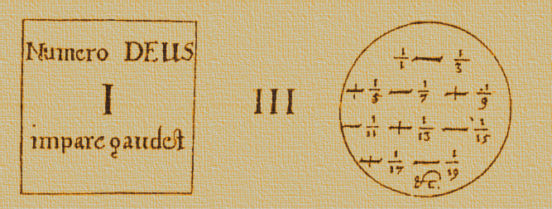
where we have used the formula for the sum of a geometric series. Leibniz comments this result with a citation from Virgil:
Numero DEUS impare gaudet (God loves the odd number).
Leibniz, although living more than 300 years ago, made important contributions to computer science. He invented a mechanical calculator and worked on the binary numeral systems. He used numbers, especially the digits 0 and 1, to illustrate the Christian concept of a creation ex nihilo:
Wie denn dergestalt die Zahlen gleichsam als in einem Spiegel die Schöpfung oder den ursprung der dinge aus Gott und sonst nichts darstellen, und also dienen solches denen heyden unbekande geheimniss aus dem Licht der vernunft einiger massen zu erclären und anzudeuten, wie unrecht die heidnische philosophi, die materi als einen mitursprung, Gott gleichsam an die Seite gesezet.
With this sentence, Leibniz touches on the still debated question, whether a person (“God”) or matter and energy is the ultimate reality. He argues for the former using the principle of sufficient reason:
Maintenant il faut s’élever à la métaphysique, en nous servant du grand principe, peu employé communément, qui porte que rien ne se fait sans raison suffisante, c’est-à-dire que rien n’arrive sans qu’il soit possible à celui qui connaîtrait assez les choses de rendre une raison qui suffise pour déterminer pourquoi il en est ainsi, et non pas autrement. Ce principe posé, la première question qu’on a droit de faire sera : pourquoi il y a plutôt quelque chose que rien ?
In spite of contrary claims, there is no convincing solution, which shows how something can come into being out of nothing. For many proposed solutions, “nothing” turns out not to be literally nothing, but for example a quantum vacuum as in the case of Lawrence Krauss. In another prominent case, the Hartle-Hawking cosmology, the universe turns out to be an eternally existing de Sitter space. A de Sitter space is a space-time with a constant positive curvature. This leads to the unusual fact, that the ratio of a circle’s circumference to its diameter is smaller than π.
This finally brings us back to π. Of course, you could use the Leibniz series to compute π numerically, but the convergence is really slow. Let’s give it a try:
Result:
1e+00 terms: 4.0
1e+01 terms: 3.0418396189294032
1e+02 terms: 3.1315929035585537
1e+03 terms: 3.140592653839794
1e+04 terms: 3.1414926535900345
1e+05 terms: 3.1415826535897198
1e+06 terms: 3.1415916535897743
As you can see, to get one more digit, you have to sum up ten times more terms. So that’s not the way to go for a new world record.




Comments by Pipi